Annals of Mathematics and Physics
Dualistic relativity: Unification of Einstein’s Special Relativity and de Broglie’s Matter–Wave Theory
Xiaogang Ruan*
Cite this as
Ruan X (2022) Dualistic relativity: Unification of Einstein’s Special Relativity and de Broglie’s Matter–Wave Theory. Ann Math Phys 5(1): 055-067. DOI: 10.17352/amp.000040Copyright Licence
© 2022 Ruan X. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited.In Hawking’s view physics has been broken up into many partial theories, while the ultimate goal of physicists is to unify them. The two basic theories of 20th-century physics, relativity theory and quantum theory, are based on completely different logical prerequisites and exactly separate: matter is described as particles in relativity theory and as waves in quantum mechanics. Here, based on the identical logical prerequisites, we unify Einstein’s special relativity (SR) and de Broglie’s matter-wave theory (MWT) into the theory of dualistic relativity (DR), taking a significant step toward the unification of relativity and quantum mechanics. From the definition of time, we derive the Lorentz transformation in differential form and establish the theory of DR, which generalizes the wave-particle duality of matter motion, and uniformly derives Einstein’s formula E=mc2, Planck’s equation E=hf, and de Broglie’s relation λ=h/p. From the logical prerequisite completely different from Einstein’s hypothesis of the invariance of light speed and along the logical path completely different from Einstein’s SR, we have deduced the whole theoretical system of Einstein’s SR and de Broglie’s MWT. In the theory of DR, the two great formulae originally separated, Einstein’s formula E=mc2 and Planck’s equation E=hf, become a pair of twin formulae unified in an identical theoretical system.
Introduction
Over the past 100 years, the wave-particle duality (WPD) of matter motion has been the main focus of modern physics. However, to this day, the image of the WPD is still not very clear in physics; the particle and wave natures of matter motion still have to be formalized by different theoretical systems. As Einstein and Infeld remarked [1], “It seems as though we must use sometimes the one theory and sometimes the other, while at times we may use either.” In Hawking’s view [2], the two basic theories of 20th-century physics, relativity theory, and quantum theory are completely separate: matter is described as particles in relativity theory and as waves in quantum mechanics. Hawking had always attempted to unify relativity and quantum mechanics when he was alive [3,4].
Relativity theory and quantum theory are established based on completely different logical prerequisites or axioms, which is why they are separate from each other. Two hypotheses play crucial roles in relativity theory and quantum theory: one is Einstein’s hypothesis of the invariance of light speed (ILS) not only for special relativity (SR) [5] but also for general relativity (GR) [6]; the other is Planck’s quantum hypothesis of E=hf for quantum mechanics [7,8].
In 1887 following Maxwell’s proposal [9] Michelson and Morley experimented to search for the ether [10]. They failed to catch the ether but found that the speed of light plus the orbital speed of the Earth remained the speed of light. To interpret the Michelson–Morley experiment FitzGerald proposed the hypothesis that space contracts by a factor of √(1−v2/c2) along the line of motion [11]. Later, Lorentz added the hypothesis that time dilates by a factor of 1/√(1−v2/c2) [12]. Thus, the Lorentz (or FitzGerald–Lorentz) transformation was born. In 1905 Einstein proposed the ILS hypothesis, based on which he theoretically derived the Lorentz transformation, and then established the whole theoretical system of SR5.
In 1900 Planck proposed the quantum hypothesis: E=hf and then theoretically derived the law of blackbody radiation [7] that coincides well with the experimental results of blackbody radiation. However, what matters most seems to be not the law itself, but its hypothetical proposition: E=hf, which implies that the energy of light is discrete rather than continuous, and thereby, lays the first cornerstone of quantum theory. In the 1920s de Broglie proposed the WPD hypothesis [13,14]: any matter particle has its wavelength and behaves as a wave just like photons, based on which he extended Planck’s equation E=hf from photons to all matter particles, derived the de Broglie relation λ=h/p, and established his matter–wave theory (MWT) [15-17]. de Broglie’s MWT is an important link in the formation of quantum theory, and it lays the second cornerstone of quantum theory. Inspired by de Broglie’s MWT, Schrödinger conceived a wave equation, that is, the famous Schrödinger equation [18] that lays the third cornerstone of quantum theory.
In a sense, a partial theory is an incomplete theory. Einstein, et al. always questioned the completeness of quantum mechanics [19]; while Einstein’s SR seems to be incomplete as well due to the Lorentz singularity, at which |v|=c and the Lorentz factor γ=1/√(1−v2/c2) reaches infinity. Hawking said [2], “Mathematics cannot really handle infinite numbers. A theory itself breaks down at a point called a singularity by mathematicians.” According to the mass–velocity relation of SR, if |v|=c, the relativistic mass m is infinite: m=γmo=∞, unless the rest mass mo is zero; in which case m is indefinite. In Hawking’s words, Einstein’s SR breaks down at the Lorentz singularity. It follows that the relativistic masses of photons cannot be determined by relativity theory itself and has to be calculated by means of Planck’s quantum hypothesis: let mc2=hf, then m=hf/c2. E=mc2 and E=hf belong to different theoretical systems; so why does mc2=hf?
Both relativity theory and quantum theory are the mathematical models of matter motion, involving the different aspects of the WPD of matter motion, and they should logically share the common axiomatic system. In this paper by taking the definition of time as the most basic prerequisite, we establish the theory of dualistic relativity (DR), generalizing the WPD of matter motion and unifying Einstein’s SR and de Broglie’s MWT. In the theory of DR Einstein’s formula E=mc2, Planck’s equation E=hf, and de Broglie’s relation λ=h/p can be uniformly derived; Einstein’s ILS and Planck’s E=hf are no longer hypotheses, but logical inferences derived in theory, and as de Broglie would like, Planck’s equation E=hf theoretically extends to all matter particles, not merely used for photons. The theory of DR suggests that the Lorentz transformation can be derived from more basic prerequisites than Einstein’s ILS hypothesis; Planck’s blackbody radiation law can be derived from more basic prerequisites than Planck’s quantum hypothesis. Different logical prerequisites and different logical paths deduce the same things, which corroborates the logical rationality and self-consistency of DR theory from one aspect. In particular, with the common logical prerequisites, Einstein’s SR and de Broglie’s MWT have been integrated into an identical theoretical system.
Time and its measurement
Time is the most basic physical concept, or the most basic physical quantity, and plays a crucial role in both the relativistic effects and quantum effects of matter motion.
Definition of time: The measurement of time depends on periodic matter motion. In theory, any periodic phenomena can be employed to measure time [20]: its period can be defined as the basic unit of time. In the International System of Units (SI) the basic unit of time is defined as the period of the radiation corresponding to the transition between the two hyperfine levels of the ground state of the cesium-133 atom [21]: 9,192,631,770 successive such periods make a second. Note that this definition requires the cesium-133 atom to be in a free spacetime and static with respect to the observer, which implies that time may be different in different spacetime environments or relative to different observers.
de Broglie’s WPD hypothesis [13,14] suggests that matter particles behave like waves. Waves have an important property: periodicity, and therefore possess the special capacity to measure time. In the WPD sense, any observed object is a natural clock, what is called a matter-wave clock, as Sanders remarks [22]: “A rock is a clock.” Müller, a professor at the University of California, Berkeley, USA, introduced his research on matter-wave time and matter-wave clock in Nature magazine (2010) [23] and at an international conference (2013) [24] and claimed that in the future matter-wave clocks would perhaps be more accurate than atomic clocks.
Of course, a practical matter–a wave clock is not essential for the theory of DR: what DR needs is just the concept or definition of time that can reflect the essence of time.
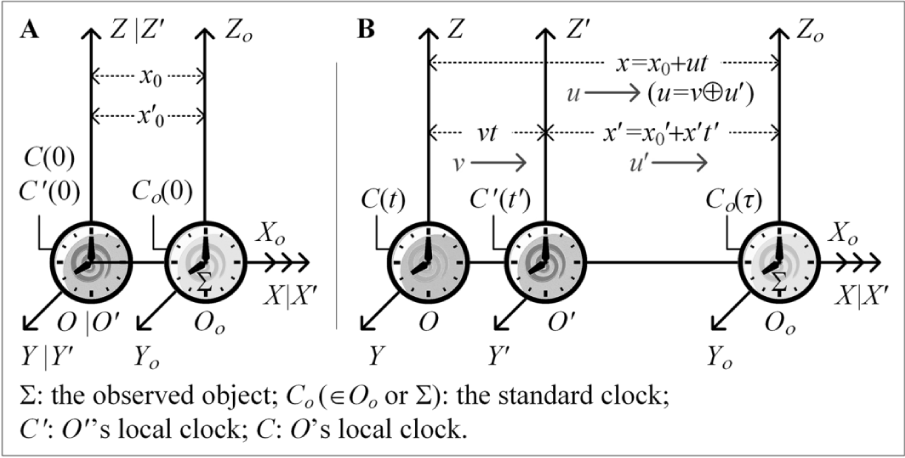
In this study we agree that (i) Oo stands for the free spacetime where there is no force or interaction; (ii) the intrinsic period of a periodic signal is its period when the signal source and the observer are both at rest in Oo; (iii) Σ stands for the observed object, and its coordinate is identically the origin of Oo, so that Oo serves as the intrinsic reference frame of Σ, and at times, also denotes the intrinsic observer of Σ.
The hypothesis of WPD is still a basic prerequisite for DR and is restated below.
The hypothesis of WPD: Any observed object behaves both as a particle and as a wave (called a matter-wave), and therefore, has its own intrinsic period, frequency, and wavelength.
Under the hypothesis of WPD Σ is a clock equivalent to the periodic motion of Σ’s matter-wave. Let To be Σ’s intrinsic period, and fo (=1/To) Σ’s intrinsic frequency, then To can be employed as the basic unit for measuring time, and time can be defined with Σ’s To or fo.
Definition A (time): Let O be an observer of Σ. If O detects N periods of Σ as the duration of ∆t, then ∆t=NTo=N/fo. If O is Oo, ∆t is called the intrinsic time of and denoted by ∆τ (=NoTo =No/fo), where No is the number of Σ’s periods detected by Oo for the duration of ∆τ; otherwise, ∆t is called the observed time of O.
Invariance of time-frequency ratio
Definition A suggests that different observers have observed different times, and yet also implies a sort of temporal invariance.
The free spacetime Oo can be regarded as the intrinsic observer of Σ; in addition, let O and O′ be any two observers observing Σ independently. As depicted in Figure 1, Oo, O, and O′ have different observed periods and different observed frequencies, and therefore have different observed times; however, they must follow the common criterion for time. The observed object Σ serves as the standard clock in Definition A; logically, its intrinsic quantities (including To, fo, and τ) can be employed as the criteria for time. In Definition A the intrinsic period To and the intrinsic frequency fo are taken as the criteria for defining time. As shown in Table 1, the respective observed periods and frequencies of Oo, O, and O′ should be determined by the intrinsic time ∆τ.
According to Definition A, the observed frequency of Oo is exactly the intrinsic frequency: fo=No/∆. Correspondingly, as shown in Table 1, the observed frequency of O should be f=N/∆τ, and f/fo=N/No=∆t/∆. Similarly, the observed frequency of O′ should be f′=N′/∆τ, and f′/fo=N′/No=∆t′/∆τ. Let ∆→d, we then get Eq. (1):
Where dt and dt′ are respectively the observed time elements of O and O′, and dτ is the intrinsic time element of Σ.
Equation (1) is an important inference from Definition A, which reveals an important property of time measurement (or observation): the invariance of the time-frequency ratio (ITFR). Equation (1) can be stated as a fundamental principle inferred from the definition of time as described below.
The principle of the ITFR: For any observer O the ratio of its observed time element dt to its observed frequency f is an invariant and identically equal to the ratio of the intrinsic time element dτ to the intrinsic frequency fo.
It is exactly the ITFR that leads to both the relativistic effects and quantum effects.
In Definition A, the observed object Σ serves as the standard clock, and its intrinsic period To or frequency fo is taken as the criterion for defining time, involving the intrinsic time τ of Σ and the observed times of different observers (Oo, O, and O′). Logically, the intrinsic time ∆τ of Σ should be taken as the criterion for determining the observed periods and observed frequencies of different observers.
Spacetime transformation
Galileo [25] and Newton [26] claimed that space and time are absolute: space is eternal and time flows quietly. The absolutist view of space and time is embodied in the Galilean transformation, in which space and time are mutually independent. Mach [27] and Einstein [28] held the relativist view that is embodied in the Lorentz transformation [11,12], where space and time are interdependent and can be transformed into each other: space is time and time is space. Thus, the unity of space and time forms the concept of spacetime [29].
Different observers represent different reference frames, while different frames mean different spacetimes or observed spacetimes including observed spaces and observed times. Therefore, spacetime transformation involves not only the transformation of different observed spaces (3d coordinates) but that of different observed times as well.
Inertial spacetimes: Similar to the Galilean and Lorentz transformations, DR examines the transformation between inertial spacetimes. The free spacetime Oo(Xo, Yo, Zo, Co) shown in Figure 1A must be the intrinsic inertial spacetime of the observed object Σ; thus, all inertial observed spacetimes of Σ can be defined on the basis of Oo.
As an observer of Σ, Oo must be equipped with its own ruler for measuring space and its own clock for measuring time. As depicted in Figure 1A, the ruler Ro of Oo is defined as 3d Cartesian coordinates: (Xo, Yo, Zo); the clock Co of Oo is defined by Definition A as a matter-wave clock equivalent to the periodic motion of Σ’s matter-wave. All observers must follow the common criterion on space and time, and be equipped with the same ruler (so-called the standard ruler) and the same clock (so-called the standard clock) so that different observers can communicate with one another, and different observed spacetimes can be transformed into one another. In DR all observers take Ro as the standard ruler and Co as the standard clock.
Let O(X,Y,Z,C) and O′(X′,Y′,X′,C′) be Σ’s two inertial spacetimes defined relative to Oo. For the ease of the following description, we assume that (as depicted in Figure 2):
(1) Σ moves at speed u in O along the X-axis, and u in O′ along X.
(2) O′ moves at speed v in O along the X-axis; or, O moves at speed −v in O′ along X′.
(3) At t=t′=0, the corresponding coordinate axes and origins of O and O coincide.
DR attempts to establish based on the ITFR the transformation relation between O and O′: O′→O (from O′ to O) and O→O′ (from O to O′).
As far as inertial motion is concerned, without loss of generality, Σ is assumed to move on the X (X′) axis and have no relative motion in the directions of the Y (Y′) and Z (Z′) axes, so that the local observers and the local clocks of Oo, O, and O′ can be assumed to be located at their respective origins (see Figure 2A). Therefore, Oo, O, and O′ sometimes stand for their respective local observers; in particular the relative motion among Oo (Σ), O, and O′ can be regarded as the relative motion among their respective local clocks (Figure 2B).
Observed STI and velocity addition
The transformation between spacetimes depends on observation; the purpose of observation is to pick up the spacetime information (STI) of observed objects, involving spatial and temporal information. The transformation between spacetimes, no matter O→O′ or O′→O, has to involve the problem of STI transmission: the STI of Σ must be transmitted to O and O′ so that Σ can be observed by O and O′; O and O′ must exchange their STI so that their space and time coordinates can be transformed into each other.
Einstein preferred to expound his theory of relativity by means of thought experiments where he would employ light beams to transmit the STI of observed objects. In fact, Einstein’s theory of relativity (including SR and GR) implies an assumption that light or electromagnetic interaction is the medium of transmitting STI.
The relative motion of a passenger, a train, and a platform is a common example of velocity addition. The passenger Σ walks in the train at the speed u′, and the STI of Σ needs to be transmitted by means of light to the observer O′ at rest in the train. The train moves at the speed v relative to the platform, and the STI of O′ must be transmitted by means of light to the observer O at rest on the platform. Regardless of relativistic effects, the speed u of Σ relative to O can be computed with Galileo’s velocity-addition law: u=u′+v, where “+” can be called Galileo’s velocity-addition operator. However, due to relativistic effects, velocity addition does not strictly follow Galileo’s law.
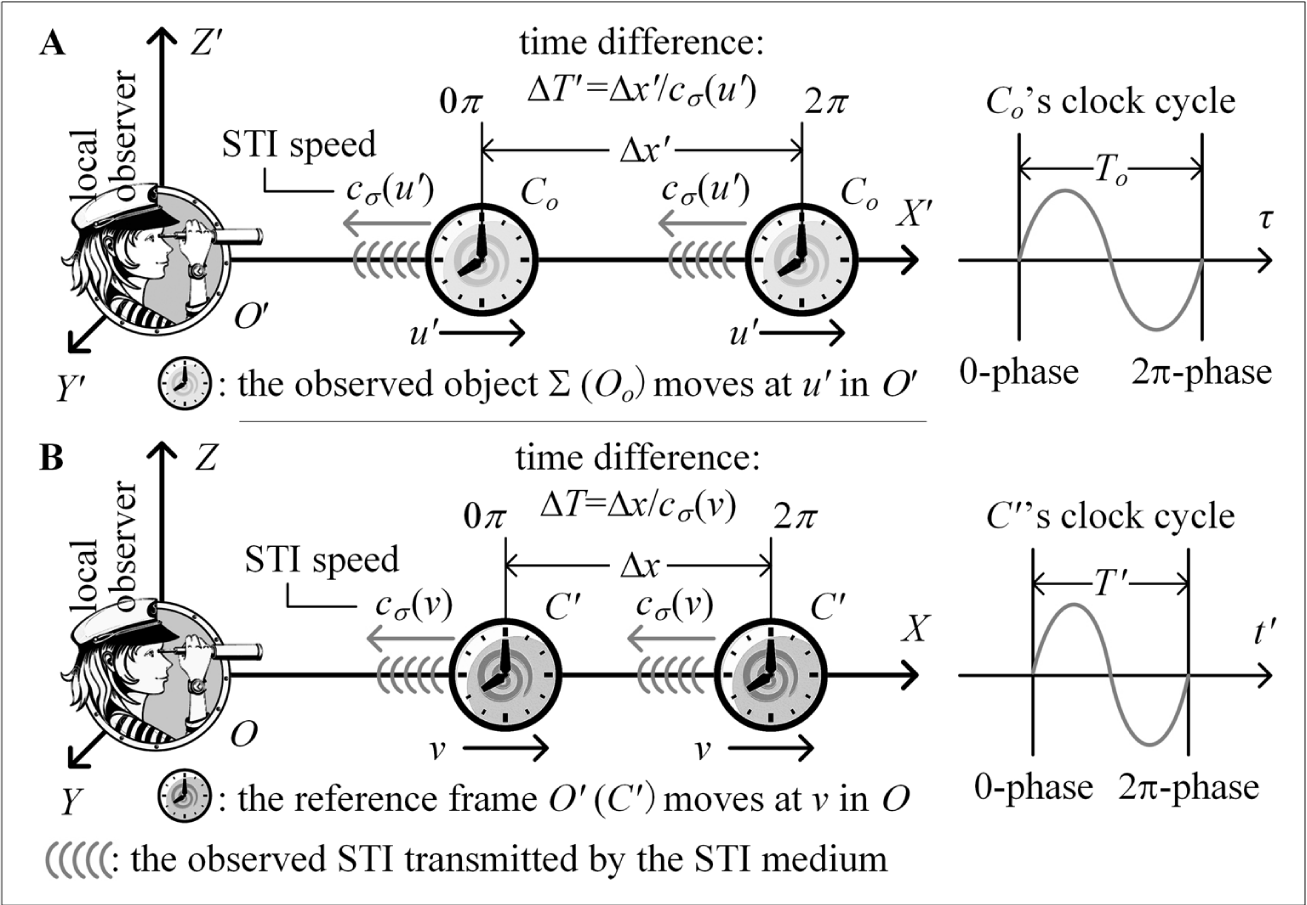
By taking relativistic effects into account let “” be the relativistic velocity-addition operator, then the relativistic velocity-addition law can be defined as u=u′⊕v. In this paper, we still suppose that light or electromagnetic interaction is the medium of transmitting STI, and its speed is the speed c of light in a vacuum. However, we do not know if the speed of light is invariant; therefore, we have to examine the velocity-addition problem of STI transmission. Logically, the STI speed relative to the observer (or the STI receiver), cσ(•), depends on the speed c of light and the speed (•) of the STI emitter relative to the STI receiver. As depicted in Figure 3, the speed c of light as the STI medium must point to the observer, and the STI speed relative to the observer should be the relativistic velocity addition of c and (•), thus we have Eq. (2):
Where if the STI emitter moves toward the STI receiver (i.e., the observer), then “⊕” is the positive-direction addition, and Eq. (2) should take “+”; otherwise, “⊕” is the negative-direction addition and Eq. (2) should take “−”.
Temporal transformation
In Einstein’s, SR the relativistic effects are rooted in Einstein’s hypothesis of the ILS, and the deduction of spacetime transformation starts from spatial transformation. In DR, however, the relativistic effects are rooted in the invariance of the time-frequency ratio (ITFR), and the deduction starts from temporal transformation.
According to the ITFR, if O′ and O directly or independently observe Σ, then we have Eq. (3):
where f(u) and f(u′) are the respective observed frequencies of O and O′, and depend on the inertial speeds u and u′, respectively. However, during the process of O′→O (O→O′), O’s (O′’s) observation of Σ is not independent and direct, which depends on O’s (O’s) observation of Σ.
First, we examine the transformation O′→O: how O observes Σ through O′.
As depicted in Figure 2, the relative motion among Oo (), O′, and O can be regarded as that among their respective local clocks Co, C′, and C. As an inertial frame, O′ can be regarded as the free spacetime of C′. According to the ITFR (Eq. (1)), when O observes O′ (C′), we have Eq. (4):
Where f(v) is the observed frequency of O observing O′’s clock C and depends on the relative speed v between O and O′; f(0) is the observed frequency of O′ observing its own clock C′ and is equal to the intrinsic frequency fo, that is, f(0)=fo.
In the transformation O′→O the STI of Σ must transmit from Σ to O′ and the STI of O′ must transmit from O′ to O, so that Σ’s motion relative to O′ can be transformed into Σ’s motion relative to O.
Each period of a wave has different phases from 0 (the start) to 2π (the end). As depicted in Figureure 1A, since Σ is at rest in Oo, the different phases of Σ’s matter-wave (or Co’s clock cycle) take the same time to travel from Σ to Oo (or the local observer of Oo). However, as depicted in Figure 4A, due to Σ moving in O′, the different phases of Co’s clock cycle take different times to travel from Σ to O′; as depicted in Figure 4B, due to O′ moving in O, different phases of C′’s clock cycle take different times to travel from O′ to O. Similarly, the start and end phases of dτ take different times to travel from Σ to O′, and that of dt′ takes different times to travel from O′ to O.
As depicted in Figure 4, we divide the process of the transformation of O′→O into two sections: (i) Σ’s STI is transmitted from Σ to O′; (ii) O′’s STI is transmitted from O′ to O.
The first section (Figure 4A): Σ moves at u′ in O′ and Σ’s STI travels from Σ to O′.
According to Eq. (2), by taking the effect of velocity-addition into account, the STI speed of relative to O′ should be cσ(u′). In the duration of O′’s observed time element dt′, Σ moves at u′ along the X′ axis a distance: dx′=u′dt′. Therefore, as depicted in Figure 4A, the start and end phases of Σ’s intrinsic time element dτ take different times to travel from Σ to O′, and the time difference is given by Eq. (5):
In the second section (see Figure 4B): O′ moves at v in O and O′’s STI travels from O′ to O.
Similarly, the STI speed of O′ relative to O should be cσ(v). From the view of O, according to Eq. (4), the observed time difference Δdt′ of O′ should be (f(v)/fo)Δdt′, during which O′ moves at v along the X-axis a distance: Δx= v(f(v)/fo)Δdt′. Therefore, as depicted in Figure 4B, the time difference Δdt′ in O′ is transformed into the time difference Δdt in O as given by Eq. (6):
Thus, according to Eq. (4) and taking into account the time difference Δdt generated in the process of the transformation O′→O, the observed time element dt of O should be given by Eq. (7):
Where γ(v)=f (v)/fo is called the relativistic factor.
Second, we examine the transformation O→O′: how O′ observes Σ through O.
In the transformation O→O′ the STI of Σ must be transmitted from Σ to O and the STI of O must be transmitted from O to O′, so that Σ’s motion relative to O can be transformed into Σ’s motion relative to O′. In the same way as O→O′, we obtain Eq. (8):
Where γ′(v)=f ′(v)/fo, f ′(v) is the observed frequency of O′ observing O (C) and depends on the relative speed v between O and O′.
Spatial transformation
Equations (7) and (8) represent the temporal transformation between the inertial spacetimes O and O′, that is, that between the observed times of O and O′, in which however they involve not only the time elements dt and dt′, but also the space elements dx and dx′. This suggests that the observed space and time of inertial spacetime are interdependent.
By combining Eqs. (7) and (8) we get the spatial transformation between O and O′ as given by Eqs. (9) and (10):
Where Γ(v)=γ(v)cσ(u)/cσ(u′) and Γ′(v)=γ′(v)cσ(u′)/cσ(u).
Equations (7)–(10) constitute the transformations between the inertial spacetimes O and O′. However, the transformation factors, γ(v), γ′(v), Γ (v) and Γ (v), have not yet been determined.
Invariance of light speed
Einstein’s ILS principle is a hypothesis, and we do not understand exactly why the speed of light is invariant. The transformation (Eqs. (7)–(10)) of inertial spacetime implies the ILS. Under the principle of relativity [30], we can theoretically deduce the ILS from Eqs. (7)–(10). Here, the principle of relativity is simply stated according to Galilean invariance [31].
The principle of relativity: Inertial spacetimes are symmetric, all inertial observers or inertial reference frames are equal in status, and therefore, the laws of physics have the same form in all inertial spacetimes.
Under the principle of relativity, O to O′ are symmetric, which requires that:
(1) in the temporal transformation Eqs. (7) and (8) γ(v)=γ′(v), that is, f(v)=f′(v);
(2) in the spatial transformation Eqs. (9) and (10) Γ(v)=Γ′(v), and hence, cσ(u)=cσ(u′).
Thus, due to the arbitrariness of u and u′, the equality as given by Eq. (11) holds:
Equation (11) suggests that the speed of light plus an inertial speed remains the speed of light. In other words, the speed of light is invariant. Thus, in DR, the ILS is no longer a hypothesis, but a logical inference derived from the ITFR and can be stated as a physical law.
The law of ILS: If light or electromagnetic interaction is employed as the medium to transmit observed information, then the speed of light is invariant, that is, the same relative to all inertial observers.
Relativistic factor
In Eq. (7), the relativistic factor is defined with γ(v)=f (v)/fo, which can be determined by Eq. (11) or the law of ILS.
Due to γ(v)=γ′(v)=Γ′(v)=Γ(v), by combining the temporal transformation Eq. (7) and the spatial transformation Eq. (9), we have Eq. (12):
According to the law of ILS or Eq. (11), cσ(u)=cσ(u′)=cσ(v)=c; if u′=c then u=u′⊕v=c⊕v=c. Thus, from Eq. (12) we get Eq. (13):
As a result, the relativistic factor γ(v) has two forms in DR:
(1) the waveform: (v)=f (v)/fo that can be called the de Broglie factor;
(2) the particle form: (v)=1/√(1−v2/c2) that is exactly the Lorentz factor.
By contrasting Eq. (13) with Eq. (1) the relativistic factor can also be formed as the ratio of the observed time element dt to the intrinsic time element dτ: γ(v)=dt/dτ, conforming to the conclusion of Einstein’s SR and GR, which from one aspect reflects the validity of not only Eq. (13) but also the ITFR and Definition A.
Lorentz transformation in differential form
In the derivation of Eqs. (7)–(10), as shown in Figure 2, Σ is located on the X (X′) axis at t=t′=0 and moves along the X (X′) axis at t>0 (t′>0), and the local observer and clock of O (O′) is located at the origins of O (O′).
In general we suppose that Σ is located at (x0,y0,z0) in O ((x0′,y0′,z0′) in O′) at t = t′ = 0 and moves in the direction parallel to the X (X′) axis. Then we imagine that the local observer and clock of O (O′) is located at (0,y0,z0) in O ((0,y0′,z0′) in O′), so that O’s (O′’s) observation of Σ remains an inertial observation and Eqs. (7)–(10) still hold. Since Σ has no relative motion in the directions of the Y (Y′) and Z (Z′) axes, it holds that dy=dy′=0 and dz=dz′=0. Thus, by substituting γ(v) for Γ(v), Γ′(v), and γ′(v) in Eqs. (7)–(10), and c for c(u), cσ(u′), and csym
(v), we have the Lorentz transformation in differential form given by Eq. (14):
Eq. (14) is the differential form of the Lorentz transformation, which is based on more basic logical prerequisites, and, hence, has more general significance than the algebraic form, and may provide new insight into the Lorentz transformation.
Particle kinematics
In essence, Einstein’s SR is particle kinematics based on the Lorentz transformation. Now we can reach the Lorentz transformation by solving the differential equation group (Eq. (14)), and then, by following Einstein’s logic, we can establish the particle kinematics of DR, generalizing the whole theoretical system of SR and deriving all the kinematic and dynamic relations of SR. Here we examine the part related to the matter-wave kinematics of DR, mainly involving the relativistic velocity addition, mass–velocity relation, and Einstein mass-energy equation.
Lorentz transformation in algebraic form
Set the initial conditions: at t=t′=0 the observed object Σ is located at (x0,y0,z0) in O ((x0′,y0′,z0′) in O′) where x0=x0′, y0=y0′, and z0=z0′ since the corresponding coordinate axes and origins of O and O′ coincide.
Then, by integrating Eq. (14) we get the Lorentz transformation in the algebraic form as given by Eq. (15):
Which is exactly the Lorentz transformation derived by Einstein from the ILS hypothesis, which is originally the phenomenological model conceived by FitzGerald [11] and Lorentz [12] according to the Michelson–Morey experiment [10]. But now the Lorentz transformation (Eq. (15)) is only a special solution of the differential form (Eq. (14)).
It is worth noting that the Lorentz transformation, no matter the differential or the algebraic representation, is not the transformation of the corresponding coordinates between O and O′. It is the transformation between the spacetime coordinates (x,y,z,t) of Σ in O and the (x′,y′,z′,t′) of Σ in O′, where the inertial observers of must be located on the line of Σ’s motion. From the view of inertial observers Σ has no relative motion along with the Y (Y′) and Z (Z′) axes, and therefore, the real meaning of y=y′ in the Lorentz transformation (Eq. (15)) is y=y(0)=y0 and y′=y′(0)=y0′, and that of z=z′ is z=z(0)=z0 and z′=z′(0)=z0′.
Relativistic velocity addition
In Einstein’s SR, the velocity-addition relation has a special status and significance, which is different from Galileo’s velocity addition. It is the phenomenon of violating Galileo’s velocity-addition law in the Michelson–Morley experiment that revealed relativistic effects and leads to the Lorentz transformation and Einstein’s SR.
Previously, we qualitatively defined the relativistic velocity addition with the operator “⊕”: u = u′⊕v. Now in DR, the relativistic velocity addition can directly be derived from the differential form (Eq. (14)) of the Lorentz transformation given by Eq. (16):
Where ux, uy, uz are the projections of Σ’s velocity u in O on the X, Y, and Z axes, and ux′, uy′, uz′ are the projections of Σ’s velocity u′ in O′ on the X′, Y, and Z′ axes.
Equation (16) seems to be exactly SR’s velocity addition, where ux=u and ux′=u′. However, it is worth noting that the inertial observation of O (O′) to Σ requires dy=dy′=0 and dz=dz′=0 in Eq. (14), which suggests that uy=uy′≡0 and uz=uz′≡0 in Eq. (16). Therefore, we should realize that SR’s velocity addition will not satisfy the prerequisite of inertial observation if uy, uz, uy′, and uz′ are nonzero, or if has relative motion along with the Y (Y′) and Z (Z′) axes.
The speeds in the relativistic speed addition (Eq. (16)) are the particle speeds of matter as particles, besides which, under the wave-particle duality (WPD) matter as waves still have their phase speeds and group speeds.
Mass–velocity relation and relativistic-momentum
The most basic relativistic relation in Einstein’s SR should be the mass–velocity relation.
Einstein introduced the concepts of rest mass and relativistic mass in his SR. According to Einstein’s SR, the observed object Σ has both its rest mass mo and its relativistic mass m. For the inertial spacetimes O and O′, let O′ be Oo, then Σ is at rest in O′, u′=0 and u=v. Thus, the mass of Σ in O is the relativistic mass m(v), depending on Σ’s speed v in O; while the mass of Σ in O′ or Oo is its rest mass mo (or called Σ’s intrinsic mass).
Naturally, the mass–velocity relation of Einstein’s SR still holds true in DR as given by Eq. (17):
Where m(v) can be called moving mass, that is, the mass of moving at speed v.
It is worth noting that the speed v in the mass–velocity relation (Eq. (17)) is the particle speed of Σ; in the wave kinematics of DR. Thus the particle speed v of Σ will be linked with the phase speed vp and the group speed vg of Σ, and the mass–velocity relation (Eq. (17)) will be linked with the frequency–velocity relation.
Correspondingly, the relativistic momentum p(v) of Σ as a moving body in O is defined as the product of Σ’s speed v and its moving mass m(v) as given by Eq. (18):
Which will be used to define the concept of force and to derive both the Einstein formula E=mc2 and Planck’s equation E=hf in a consistent way.
Mass–energy relation
Einstein’s mass-energy relation E=mc2 is the best-known formula, where m is the relativistic mass of Σ in O (when O′=Oo), depending on the speed v of Σ relative to O; E is the free energy of Σ in O. The free energy E=mc2 of Σ is composed of two parts:
(1) the rest energy of Σ in O′ (Oo): Eo=moc2;
(2) the relativistic kinetic energy of Σ in O: K=(γ(v)−1)moc2, approximately the classical kinetic energy in Newtonian mechanics if v<
Naturally, by following Einstein’s logic in SR, DR can also derive the mass-energy relation. For the purpose of making an analogy with the derivation of Planck’s equation E=hf in the following section (matter-wave kinematics), the derivation of Einstein’s mass-energy relation E=mc2 as described below is duplicated.
By defining the force on Σ in O with the relativistic momentum p=γ(v)mov (see Eq. (18)): F=dp/dt, the relativistic kinetic energy of Σ in O can be written as Eq. (19):
Where E(0)=moc2=Eo is the rest energy, while E(v) is the total energy of reaching speed v, which is given by Eq. (20):
Equation (20) is exactly the famous Einstein formula.
Matter-wave kinematics
The concept of time stated in Definition A implies the observational property of time: the ITFR. The relativistic factor γ(v) based on the ITFR links the particle and wave natures of matter motion so that DR can derive not only Einstein’s SR but also de Broglie’s MWT.
Frequency–velocity relation
By combining the two forms of the relativistic factor (see Eq. (13)), γ(v)=f(v)/fo and γ(v)=1/√(1−v2/c2), we get the frequency–velocity relation given by Eq. (21):
where fo is the intrinsic frequency of Σ’s matter-wave, and f(v) is the observed frequency in O (when O′=Oo), depending on the speed v of Σ in O.
In the theoretical system of DR the frequency–velocity relation is of important significance. Based on Eq. (21), DR can derive all the relations in de Broglie’s MWT, including Planck’s equation E=hf and the de Broglie relation λ=h/p.
Invariance of mass–frequency (energy–frequency) ratio
The frequency–velocity relation (Eq. (21)) and the mass–velocity relation (Eq. (17)) have exactly the same form. By combining Eqs. (17) and (21), we get Eq. (22):
Where hm is the mass–frequency ratio constant, that is, the ratio of the intrinsic mass (the rest mass) mo to the intrinsic frequency fo of the matter: hm=mo/fo.
Equation (22) can be stated as a principle as shown below.
The principle of the invariance of mass–frequency ratio: The ratio of the observed mass m of Σ to the observed frequency f of Σ’s matter-wave is a constant (hm) that is identically equal to the ratio of its intrinsic mass mo to its intrinsic frequency fo: hm=mo/fo.
The mass–frequency relation (Eq. (22)) implies that Eq. (23) holds:
Remarkably, Eq. (23) has exactly the same form as Planck equation E=hf.
Just as Planck’s equation E=hf implies that energy is discrete, the mass–frequency relation m=hmf implies that mass is also discrete. Perhaps, the discretization of energy is rooted in that of mass. We may say that matter is discrete with discrete mass and discrete energy.
The discretization of energy leads to the concept of a quantum (or energy quantum, meaning a portion of energy that is indivisible), and eventually to quantum mechanics (7, 8). However, the mass–frequency relation (Eq. (23)) suggests that quantum should be mass quantum, that is, a portion of mass that cannot be divided anymore.
By combining Eqs. (20) and (22), we get Eq. (24):
Where hE = hmc2 is the energy–frequency ratio constant.
Equation (24) can be started by the following principle.
The principle of the invariance of energy–frequency ratio: The ratio of the observed energy E of Σ to the observed frequency f of Σ’ matter-wave is a constant: hE=hmc2.
The energy–frequency relation (Eq. (24)) implies that Eq. (25) holds:
Equation (25) is exactly Planck’s equation E=hf where the energy–frequency ratio constant hE is exactly the Planck constant h=6.6260693×10−34 J⋅s.
Planck’s equation
Note that Eq. (25) uses Einstein’s mass-energy relation E=mc2. Actually, in the theoretical system of DR Planck’s equation and the Einstein formula are equal in status. We can derive Planck’s equation E=hEf in the same or a similar way as we derive the Einstein formula E=mc2. Using the waveform γ(v)=f(v)/fo and particle form γ(v)=1/√(1−v2/c2) of the relativistic γ, we get Eq. (26):
By following the same logic used to derive Eq. (19), then as far as Σ moving at an inertial speed v is concerned, its kinetic energy should be given by Eq. (27):
Where E(0)=hEfo=Eo is the rest energy, while E(v) is the total energy of reaching speed v as given by Eq. (28):
Equation (28) is exactly Planck’s equation. It is worth noting that Eq. (28) suggests that Σ can be any observed object. As de Broglie would expect, Planck’s equation can be used for all matter particles (even all objects), rather than merely for photons. The Planck constant h was originally the energy–frequency ratio constant of photons, but now becomes the energy–frequency ratio constant of any matter particle (even any object).
Now that Planck’s equation E=hf is no longer a hypothesis, de Broglie’s generalization has also been proven theoretically. Planck’s equation E=hf and the Einstein formula E=mc2 no longer belong to different theoretical systems and have become a pair of twin formulae of the WPD: E=mc2 is for matter particles; E=hf is for matter waves. Note that both Eqs. (19) and (27) formulize the kinetic energy of the identical observed object, which suggests mc2=hf; so that the relativistic mass m of an object can be determined by combining E=mc2 (Eq. (19)) and E=hf (Eq. (27)): m=hf/c2.
Remarkably, now that Planck’s equation E=hf has already become one of the logical consequences of DR, Planck’s law [7], the Wien displacement law [32] and the Stefan–Boltzmann law [33,34] theoretically can be derived from DR, and therefore become part of matter-wave kinematics in the theoretical system of DR.
de Broglie relation: In de Broglie’s MWT the most important content is naturally the de Broglie relation p=h/λ.
In the sense of the WPD, Σ as a matter particle has its particle speed v, and as a matter-wave its phase speed vp and group speed vg. Following de Broglie’s logic [13-15], we can get the relation between the particle speed v and the phase speed vp: v=c2/vp. Thus, based on the definition (Eq. (18)) of relativistic momentum and the relativistic factor γ(v)=f(v)/fo in wave form, we can get the de Broglie relation given by Eq. (29):
Where λ(v)=vp/f(v) is the observed wavelength of Σ’s matter-wave in O (when O′=Oo), and called the de Broglie wavelength.
In de Broglie’s MWT the de Broglie relation p=h/λ (Eq. (29)) can be rewritten as p=ħk and called the first de Broglie equation, where k=2π/λ is the wave number; the relation E=hf (Eq. (28)) extended by de Broglie can be rewritten as E=ħω and called the second de Broglie equation, where ω=2πf is the angular frequency. So we have that both the first de Broglie equation and the second de Broglie equation theoretically can be derived with the theory of DR.
Relationships among v, vp, vg: In general phase speed is defined as vp = ω/k, and group speed as vg = dω/dk. Based on the first and second de Broglie equations, the phase speed vp and group speed vg of matter waves can be redefined by energy E and momentum p with Eq. (30):
Relationships among v, vp, vg: In general phase speed is defined as vp = ω/k, and group speed as vg = dω/dk. Based on the first and second de Broglie equations, the phase speed vp and group speed vg of matter waves can be redefined by energy E and momentum p with Eq. (30):
By combining the mass–velocity relation (Eq. (17)) and the mass-energy relation (Eq. (20)), we get Eq. (31):
Thus, Eq. (32) holds for the particle speed v, phase speed vp, and group speed vg:
Perhaps we should hold the view that the particle nature of matter is the nature of the mass, the particle speed v is the transmission speed of matter mass; the wave nature of matter is the nature of energy, and the group speed vg is the transmission speed of matter-energy. Eq. (32) suggests that the transmission speed of matter mass is the same as that of matter-energy.
Conclusion
In this study, Einstein’s special relativity (SR) and de Broglie’s matter-wave theory (MWT) have been unified under the common axiom system and integrated into the identical theoretical system that is referred to as the theory of dualistic relativity (DR). The logical presuppositions of DR theory involve: (i) the hypothesis of the wave-particle duality (WPD), (ii) the definition of time, and (iii) the principle of relativity. The WPD hypothesis was proposed by de Broglie, which is in a strict sense not the prerequisite of DR theory, but just represents a philosophical concept for supporting quantum mechanics and the theory of DR. The principle of relativity can simplify the logical deduction of DR theory, but it is not a must for the theory of DR. Actually, only the definition of time is the most basic and essential logical presupposition for the theory of DR.
Time plays the most crucial role in both the relativistic effects of matter motion and the quantum effects of matter motion. Based on the definition of time, we have deduced the invariance of time–frequency ratio (ITFR), and then established the theory of DR, generalizing the WPD of matter motion. It is the ITFR that links the particle and wave natures of matter motion. From the ITFR, the Lorentz factor is derived in particle form: γ(v)=1/√(1−v2/c2), and the Lorentz transformation in differential form, while the Lorentz transformation becomes a special solution of the differential Lorentz transformation (Eq. (14)). Then, following Einstein’s logic, the whole theoretical system of SR can be derived, which formalizes the particle nature of matter motion and is referred to as the particle kinematics of DR. From the ITFR, the Lorentz factor is also derived in wave form: tsym
(v)=f(v)/fo. Then, following de Broglie’s logic, the whole theoretical system of MWT can be derived, which formalizes the wave nature of matter motion and is referred to as the wave kinematics of DR.
In the theoretical system of DR, the invariance of light speed (ILS) is no longer a hypothesis, but a logical inference of DR, so that we can understand why the speed of light is invariant. The theory of DR suggests that the ILS is not the cause of formation of relativistic effects but one of relativistic effects, and like all relativistic phenomena, is rooted in the ITFR. Planck’s E=hf is also no longer a hypothesis, but a logical inference of DR, so that we can understand why energy is discrete. Thus, Planck’s blackbody radiation law, the Wien displacement law, the Stephan–Boltzmann’s law can be derived from DR and become the matter–wave relations of DR. It is remarkable that as de Broglie would like, E=hf is theoretically extended for all matter particles by DR, rather than just for photons.
The theory of DR has uniformly derived the Einstein formula E=mc2, Planck’s equation E=hf, and the de Broglie relation p=h/λ, which originally belonged to different theoretical systems and were derived from different logical prerequisites or axioms. Planck’s equation E=hf and the Einstein formula E=mc2 have become a pair of twin formulae in the theoretical system of DR: E=mc2 is for matter particles; E=hf is for matter waves, marking the unity of the two aspects of the WPD of matter motion.
It is an indisputable fact that relativity theory and quantum theory are two completely separate theoretical systems and based on completely different logical presuppositions: the prerequisite of Einstein’s SR is the hypothesis of the invariance of light speed (ILS); while, the prerequisite of quantum mechanics is Planck’s quantum hypothesis of E=hf.
Now, from more basic prerequisite, the definition of time, the theory of DR cannot only derive the ILS, the Lorentz transformation and the whole theoretical system of Einstein’s SR, but also Planck’s equation E=hf and blackbody radiation law, and de Broglie’s MWT. From completely different logical prerequisites and along completely different logical paths, the theory of DR has deduced to the same things as Einstein’s SR, Planck’s quantum hypothesis, and de Broglie’s MWT; this corroborates the logical rationality and theoretical validity of DR theory from one aspect. More basic logical presuppositions make us not only know what the phenomena of matter motion are, but also why matter motion presents such phenomena; perhaps, this has methodological significance for physics.
Actually, the theory of DR is just one of the partial theories of observational relativity (OR for short). Based on the axiom system consisting of the definition of time, the conditions of wave-particle duality, and the principle of physical observability, we have established the theory of observational relativity, and have generalized and unified Einstein’s theory of relativity and Newton’s theory of classical mechanics. The theory of OR is composed with two parts: the first part is the theory of inertial OR [35-37] that has unified Einstein’s theory of special relativity and Galileo and Newton’s theory of inertial effects, and has generalized the general theory of matter waves, in which de Broglie’s MWT is just a special case and valid only in optical observation; the second part is theory of gravitational OR [38,39] that has unified Einstein’s theory of general relativity and Newton’s theory of universal gravitation. The interested readers can refer to the relevant literature in e-print archives or ACTA BEIJING GONGYE DAXUE [35-39].
This work was supported by Beijing High-Grade Discipline Construction Project of Control Science and Engineering.
- Einstein A. L. Infeld, The Evolution of Physics (Simon and Schuster, New York, NY). 1938
- Hawking SW. A Brief History of Time: From the Big Bang to Black Holes (Bantam Dell Publishing Group, New York, NY). 1988.
- Hawking SW. The Theory of Everything: The Origin and Fate of the Universe (New Millennium Audio, Beverly Hills, CA). 2002
- Capra F, Luisi PL. “The unification of physics,” in The Systems View of Life: A Unifying Vision. (Cambridge University Press, Cambridge). 2014.
- Einstein A. Zur Elektrodynamik bewegter Körper. Annalen der Physik. 1905; 322: 891–921.
- Einstein A. Die Grundlage der allgemeinen Relativitätstheorie. Annalen der Physik. 1916; 354:769-822.
- Planck M. Zur theorie des gesetzes der energieverteilung im normalspectrum. Verhandlungen der Deutschen Physikalischen Gesellschaft. 1990; 2: 237-245.
- Nauenberg M. Max Planck and the birth of the quantum hypothesis. Am J Phys. 2016; 84: 709–720.
- Maxwell JC. On a possible mode of detecting a motion of the solar system through the luminiferous ether. Nature. 1880; 21: 314-315.
- Michelson AA, Morley EW. On the relative motion of the earth and the luminiferous ether. Sidereal Messenger. 1887; 6: 306-310.
- FitzGerald GF. The ether and the earth's atmosphere. Science. 1889; 13: 390.
- Lorentz HA. The relative motion of the earth and the aether. Zittingsverlag Akad. v. Wet. 1892; 1: 74-79.
- de Broglie L. Waves and quanta. Nature. 1923; 112: 540.
- de Broglie L. Recherches sur la théorie des Quanta. Thesis, University of Paris, Paris. 1924.
- de Broglie L. XXXV. A tentative theory of light quanta. Philos Mag. 1924. 47: 446-458.
- Weinberger P. Revisiting Louis de Broglie's famous 1924 paper in the Philosophical Magazine. Philos Mag Lett. 2006; 86: 405-410.
- Medicus HA. Fifty years of matter waves. Phys Today. 1974; 27: 38-45.
- Schrödinger E. Quantisierung als Eigenwertproblem. Annalen der Physik. 1926. 384: 361-376.
- Einstein A, Podolsky B, Rosen N. Can quantum-mechanical description of physical reality be considered complete? Phys Rev 47: 777–780.
- Barnett JE. Time's Pendulum: From Sundials to Atomic Clocks, the Fascinating History of Timekeeping and How Our Discoveries Changed the World (Houghton Mifflin Harcourt, Boston, MA). 1999.
- Thompson EA, Taylor BN. Guide for the use of the International System of Units (SI). (NIST Special Publication, National Institute of Standards and Technology, Gaithersburg, MD). 2008.
- Sanders R. A rock is a clock: physicist uses matter to tell time. 2013.
- Müller H, Peters A, Chu S. A precision measurement of the gravitational redshift by the interference of matter waves. Nature. 2010 Feb 18;463(7283):926-9. doi: 10.1038/nature08776. PMID: 20164925.
- Müller H. Paper presented at the International School of Physics "Enrico Fermi" 2013, Course 188 - Atom Interferometry, Varenna, 15–20 July 2013.
- Drake S. Essays on Galileo and the History and Philosophy of Science (University of Toronto Press, Toronto) 1999.
- Newton I. Philosophiae Naturalis Principia Mathematica (Joseph Streater, London) 1687.
- Mach E. Die Mechanik in ihrer Entwickelung, historisch-kritisch dargestellt (F.A. Brockhaus, Leipzig) 1883.
- Einstein A. Ernst Mach. Physikalische Zeitschrift. 1916; 17: 101-104.
- Minkowski H. Raum und Zeit. Physikalische Zeitschrift. 10: 75-88.
- Lorentz HA, Einstein A, Minkowski H, Weyl H, Sommerfeld A. The Principle of Relativity: A Collection of Original Memoirs on the Special and General Theory of Relativity (Courier Corporation, North Chelmsford, MA). 1952.
- Galilei G. Dialogue Concerning the Two Chief World Systems, Ptolemaic and Copernican (Modern Library Classics, New York, NY, 2001).
- Wien W. Eine neue Beziehung der Strahlung schwarzer Körper zum zweiten Hauptsatz der Wärmetheorie. Sitzungsber. d. Berl. Akad. 1893; 55-62.
- Stefan J. Über die Beziehung zwischen der Wärmestrahlung und der Temperatur, Sitzungsberichte der mathematisch-naturwissenschaftlichen Classe der kaiserlichen. Akademie der Wissenschaften. 1879; 79: 391-428.
- Boltzmann L. Ableitung des Stefan'schen Gesetzes, betreffend die Abhängigkeit der Wärmestrahlung von der Temperatur aus der electromagnetischen Lichttheorie. Annalen der Physik. 1884; 258: 291-294.
- Ruan X. Information wave and the theory of observational relativity. viXra: 1707.0379, 2017.
- Ruan X. Observational relativity: bringing to light the essence of relativistic effects. CHINA: Sciencepaper 2018.
- Ruan X. Observation and relativity: why is the speed of light invariant in Einstein’s special relativity? Journal of Beijing University of Technology. 2020; 46: 82-124.
- Ruan X. General observational relativity: why is the spacetime curved in Einstein’s general relativity? viXra: 2204.0055, 2022.
- Ruan X. General observational relativity: why is the spacetime curved in Einstein’s general relativity? Journal of Beijing University of Technology. 2022.
Article Alerts
Subscribe to our articles alerts and stay tuned.
 This work is licensed under a Creative Commons Attribution 4.0 International License.
This work is licensed under a Creative Commons Attribution 4.0 International License.
 Help ?
Help ?

PTZ: We're glad you're here. Please click "create a new query" if you are a new visitor to our website and need further information from us.
If you are already a member of our network and need to keep track of any developments regarding a question you have already submitted, click "take me to my Query."



 Save to Mendeley
Save to Mendeley